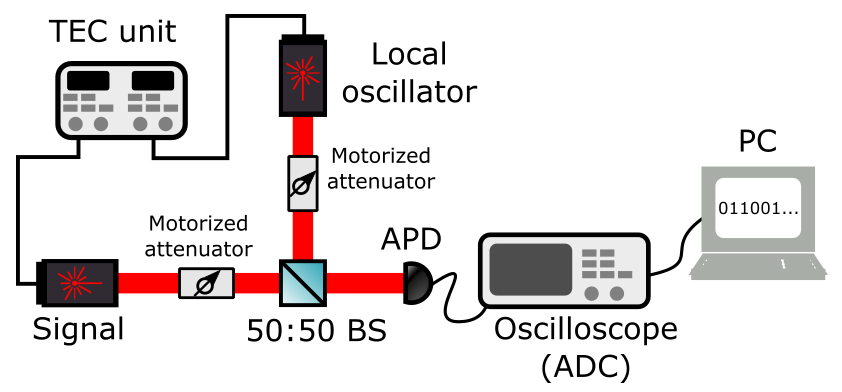
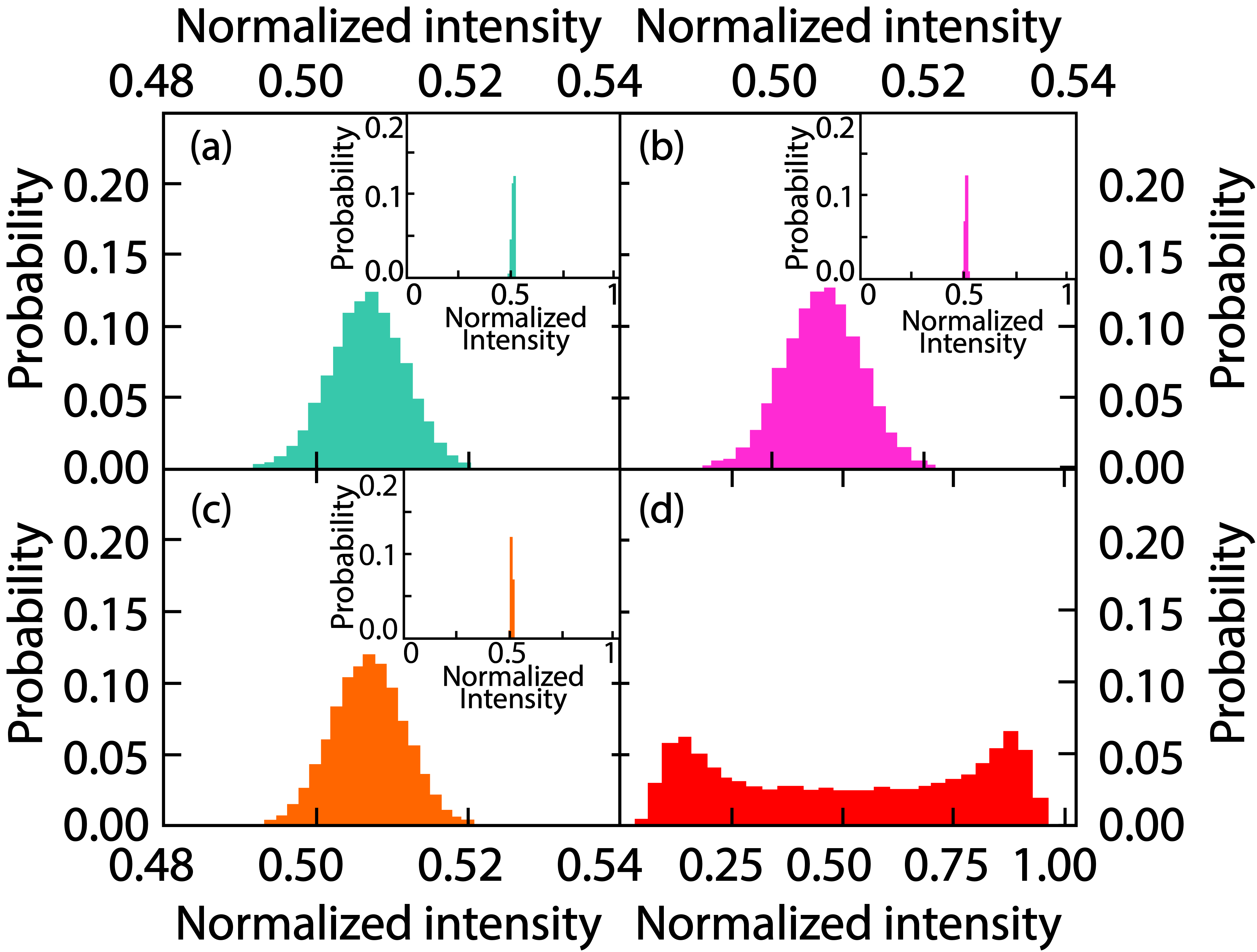
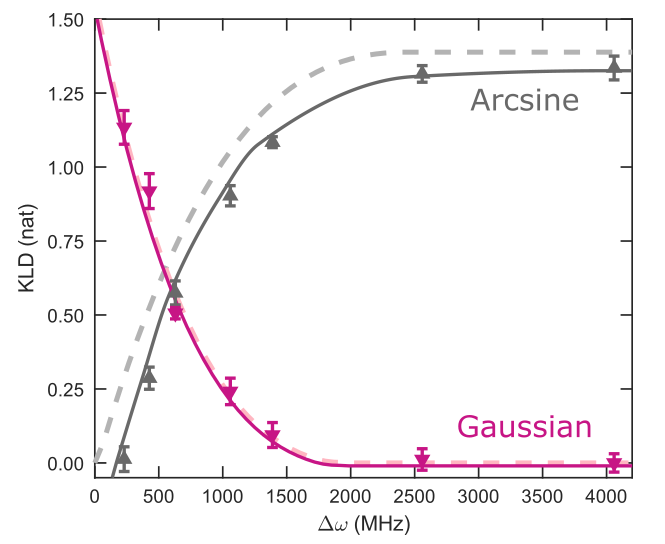
A requirement for randomness is present in many modern technologies, e.g. digital security, numerical simulations, and electronic gambling. The random numbers used in these applications must be uniformly distributed and truly independent from each other, otherwise the security of these digital systems could be compromised.
Classical algorithmic random number generators, also known as pseudo random number generators (PRNGs), are commonly used to produce random numbers, given the relative simplicity and low cost with which they can be implemented, and their comparatively fast data generation rates.
However, such generators only produce numbers which appear random when analysed using a system with limited computational power and resources. Analysis with infinite resources would reveal patterns that could be predicted. On the contrary, quantum random number generators (QRNGs), have a level of randomness which relies on the intrinsic probabilistic nature of quantum measurements dictated by quantum mechanics.
There are broad range of phenomena which can be exploited for QRNGs, for example, single-photon detection, amplified spontaneous emission noise, vacuum fluctuations, and photon number distributions.
Commercial devices, which utilise QRNGs, already exist, however, the generation rates are in the low Mbs-1 range. For applications in, for example, quantum key distribution or quantum digital signatures, which consume random numbers in the Gbs-1 regime, higher QRNG rates are required.
QRNGs that employ quantum phase noise from spontaneous emission of a laser source offer advantages in terms of generation rate, operational simplicity, and the potential to be implemented using low cost components.
Current works have shown phase randomisation from coherent states interference using asymmetric Mach‑Zehnder interferometers achieving generation rates up to Gbs-1, interference between independent lasers in different configurations and continuous variable (CV) source‑independent QRNG.
Nevertheless, randomness is an extremely fragile property and is easily lost whenever the physical system is correlated with the environment. In order to preserve such feature, it is imperative to quantify the amount of correlation and possible eavesdropping by a malicious party that would prevent the system from being used for any cryptographic purposes.
Meet the researchers
Selected publications
- U. Zanforlin, R.J. Donaldson, R.J. Collins and G.S. Buller, "Analysis of the effects of imperfections in an optical heterodyne quantum random‑number generator", Physical Review A 99, Article Number 052305 (2019).